Primary consolidation is the major part of the total settlement in soil. Primary consolidation is also known as Consolidation Settlement. It is the settlement of a soil layer due to the gradual dissipation of excess pore water pressure under a constant applied load.

When analysing the settlement of a soil layer due to consolidation, we divide the process into two main parts.

1. Computation of the magnitude of settlement
2. Determination of the time rate of settlement

For the calculation of the time rate of consolidation, Terzaghi's theory of Consolidation is used, which involves the determination of the coefficient of consolidation. We have discussed the Terzaghi’s theory of Consolidation in detail in our previous post.

For the determination of the settlement of the soil layer, we have two methods. One is based on using the coefficient of volume compressibility, and the other is based on using the Void Ratio of the soil.

Using coefficient of volume compressibility

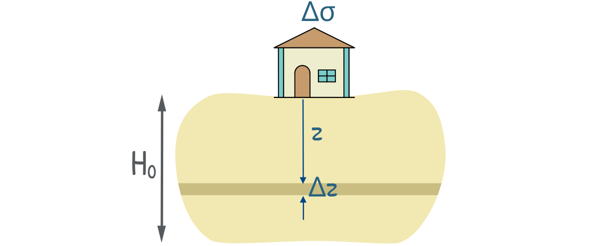
Let us consider a soil deposit of total thickness H₀. We wish to know the settlement of this soil layer, when the stress on the layer is increased by Δσ. We begin by considering a small element of thickness Δz at depth z in this soil layer.

We know that the coefficient of volume compressibility (m_v) is defined as the negative fractional change in volume of soil per unit change in effective stress acting on it: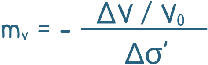

where :

• ΔV is the change in volume of soil

  V₀ is the soil’s initial volume

  Δσ’ is the change in effective stress between soil particles

Keep in mind that Δσ’ is not the change in total stress on soil, Δσ, it is change in stress between the soil particles.

We rearrange the equation for ΔV.

ΔV = - m_V V_O Δσ’

We can write the volume, as area of soil multiplied by depth in both the sides.

A ΔH = - m_V (AH_O) (Δσ’)

Now, if we assume that area of the soil layer does not change with the load increment, we can write, change in volume as change in its height.

ΔH = - m_V H_O (Δσ’)

This ΔH is the change in thickness of the soil layer, which is negative when the soil settles. However, this is, in fact, the settlement of the layer. Therefore, we can write it as S_C, i.e., settlement due to consolidation, without any minus sign.

S_C = m_V H_O (Δσ)

For the small soil element, that we considered, this settlement can be written as this

ΔS_C = m_V (Δz) (Δσ)

For the calculation of settlement of the whole layer, we can integrate it over the entire depth of the layer:

S_C = ∫ΔS_C = ∫m_V Δz (Δσ)

This is the total settlement of the soil layer.

Then, there is Void Ratio method for the determination of the settlement of the soil layer

Using Void Ratio

In this method, we consider the soil as a two-phase system, which contains only voids and soil solids. Voids may be filled by either water or air.

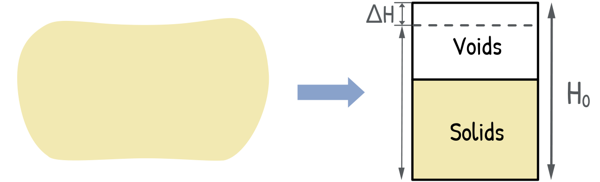
Using the two phase diagram, we can write this relationship as we consider the area of the soil to be constant.:

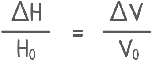
We can write total volume of soil as volume of voids plus volume of solids.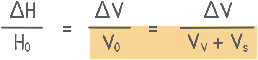

we divide both numerator and denominator by Volume of solids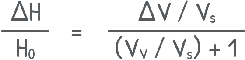

We can see the quantity, (V_V/V_s), in the denominator is void ratio and the quantity in numerator (ΔV/V_s) is change in void ratio, as we know change in volume of soil is change in its voids.

Hence, finally we get this relationship:

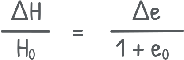
rearranging for ΔH :

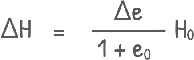
This ΔH is the consolidation settlement.

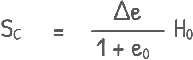
To calculate Δe (the change in void ratio of the soil due to change in pressure on the soil), we can use the plot between void ratio and log of effective stress, that we created using consolidometer test results. This test has also been discussed in our earlier post.

For soils that are experiencing the highest load in their history, known as normally consolidated soils, this curve is obtained as a straight line.

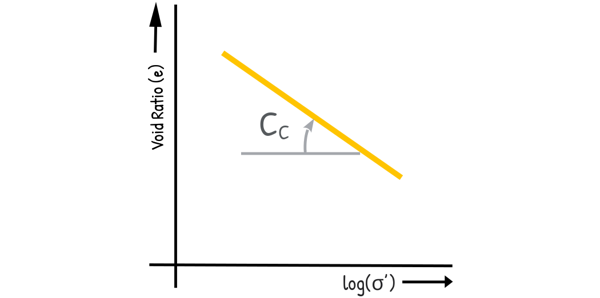
The slope of this curve is defined as the compression index (Cc) and is given as this.

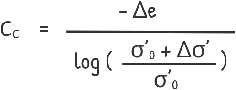
rearranging for Δe and removing minus sign for settlement:

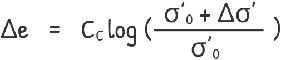
Hence, the settlement of soil becomes

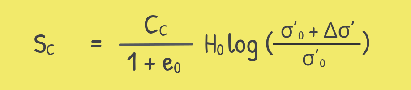
Then, for soils that have experienced a higher load in the past than they are currently experiencing, known as Overconsolidated soil, we obtain a different type of curve in the consolidometer test.

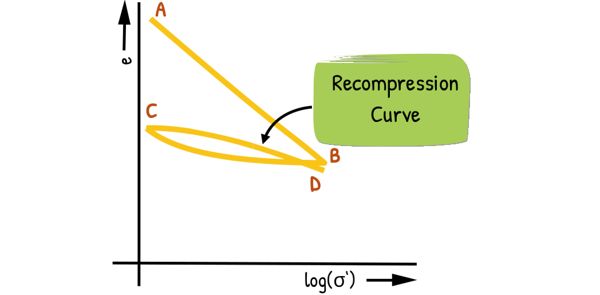
This curve is known as the Recompression curve, and the slope of this curve is referred to as the Recompression index (C_r). The recompression index can be expressed as this:

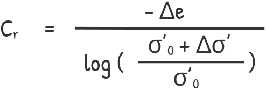
Hence, the settlement of soil becomes

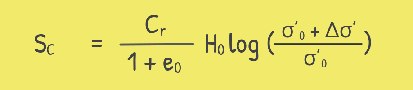
Generally, we encounter soils that have been previously subjected to a load that was later removed. Then soil is reloaded with higher load. In such cases, the soil's state changes from being overconsolidated to normally consolidated. In this scenario, we need to calculate the soil settlement in two parts:

First when soil was in oversconsolidated state, i.e. settlement when effective stress reaches from initial value to preconsolidation stress. And then, when soil is in normally consolidated state, i.e. settlement from preconsolidation stress to maximum stress.

Upto preconsolidation stress the soil remains in recompression phase, then after that soil is in normally compression phase.

So final settlement is this:

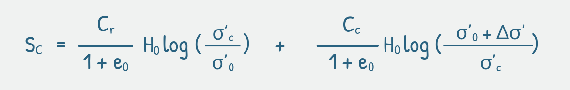
As the value of C_r is very less, value of this part is very small.

So, how are we going to calculate the settlement of soil in the field?

We begin by collecting a soil sample from the field. We use the sand replacement method for the volume determination of the soil sample. Then we oven-dry the sample, which gives us the weight of the soil solids only.

Next, we calculate the volume of the solids using the following formula:

V_S = G_S γ_W W_S

where:

• V_S is the volume of the solids,

• W_S is the weight of the solids which we determined using oven drying,

• G_S is the specific gravity of the solids (usually around 2.65-2.70 for most soils),

• γ_w is the unit weight of water.

We have the total volume of the sample and volume of soil solids, therefore, we can calculate the volume of the voids by subtracting the volume of the solids from the total volume. This allows us to calculate the initial void ratio (e₀) of the soil layer:

e₀=V_v / V_s

where:

• Vv is the volume of the voids,

• Vs is the volume of the solids.

Then, we perform a consolidometer test (oedometer test) on the soil sample to obtain the void ratio versus the log of effective stress curve. With this curve, we can determine the compression index (C_C).

Finally, we have everything we need to calculate the consolidation settlement of the soil layer using the following formula:

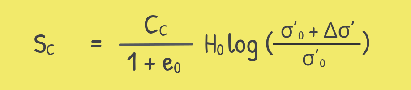
where:

• S_C is the settlement due to consolidation,

• C_C is the compression index,

• e₀ is the initial void ratio,

• H₀ is the initial thickness of the soil layer,

• σ₀′ is the initial effective vertical stress,

• Δσ′ is the increase in effective vertical stress due to the applied load.